August 2019


Non-linear IV transport: BKT physics vs inhomogeneity

One of the hallmarks of the Berezinskii-Kosterlitz-Thouless physics is the discontinuous jump of the superfluid stiffness Js at the transition temperature from a finite and universal value to zero. However, in real materials such a jump is usually replaced by a rapid and continuous downturn, that can be still ascribed to BKT physics once the low value of the vortex-core energy and a moderate sample inhomogeneity are taken into account, as our group demonstrated few years back by means of direct comparison between penetration-depth measurements and theoretical calculations (see Phys. Rev. Lett. 107, 217003 (2011)). This effect can also be evinced by means of transport measurements thanks to the possibility of a large enough current to unbind vortex-antivortex pairs below Tc, generating an extra voltage that reflects in non-linear IV characteristics. The universal jump of Js at Tc should then reflects in an universal jump of the IV exponent a from a=3 right below Tc to a=1 right above it. 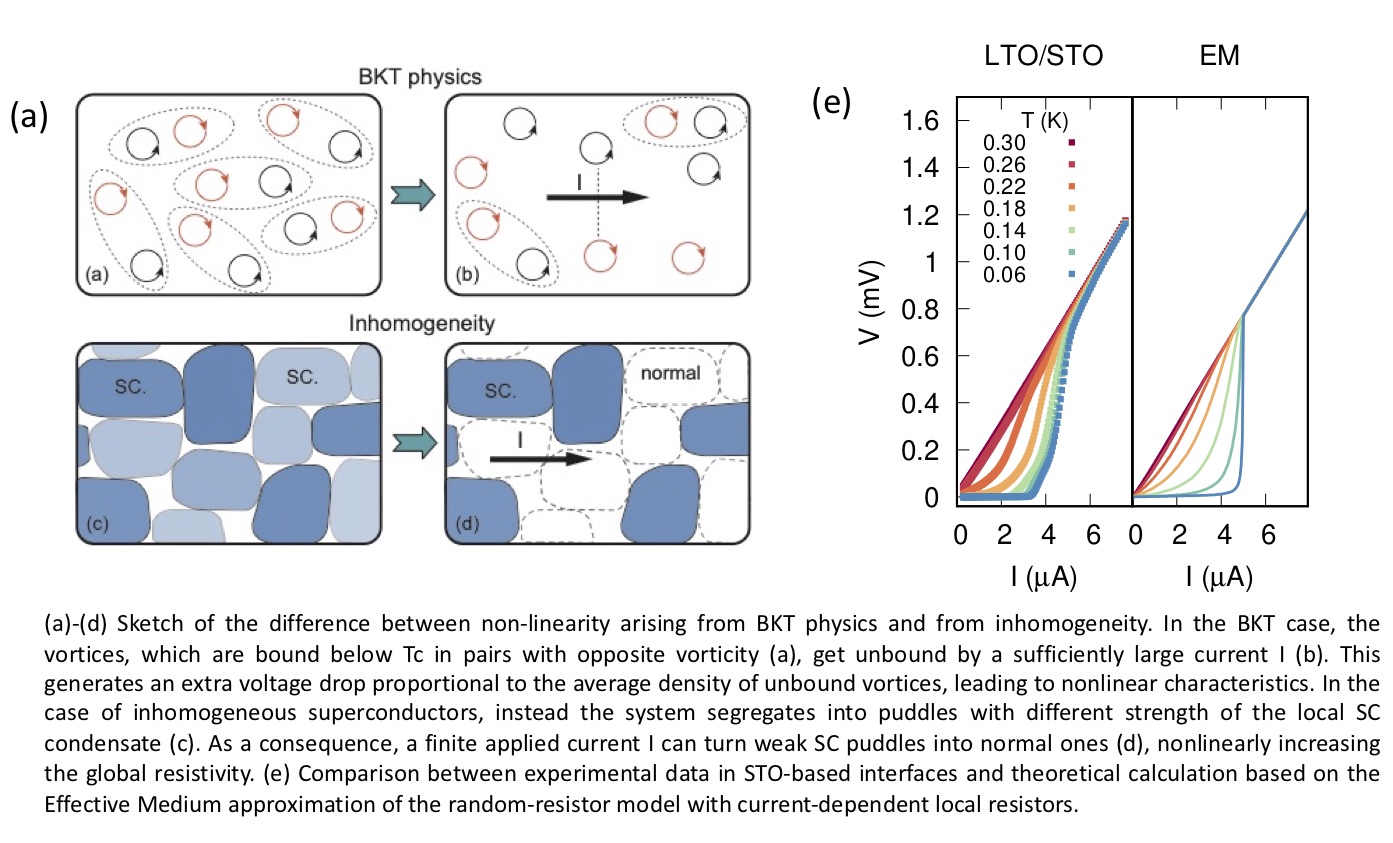What happens then when the Js jump is smeared by disorder? And what is the fate of the BKT signatures when the sample inhomogeneity occurs on mesoscopic length scales, making percolative effects more pronounced than BKT physics? In a recent work published in Phys. Rev. B 100, 064506 (2019) we demonstrated that while IV characteristics in thin NbN films represent a textbook example of BKT physics, the pronounced non linearity observed in STO-based interfaces do not seem to justify a BKT analysis. Rather, the observed I−V characteristics can be well reproduced theoretically by modeling the effect of mesoscopic inhomogeneity of the superconducting state. Our results offer an alternative perspective on the spontaneous fragmentation of the superconducting background in confined two-dimensional systems.


June 2018


Hexatic phase in MoGe thin film

According to the Berezinskii-Kosterlitz-Thouless theory, later refined by Halperin, Nelson and Young, the melting of a 2D solid crystal should happen via two subsequent transitions controlled by topological excitations. At the first transition thermally excited free dislocations proliferate in the lattice breaking the lattice rigidity but preserving its orientational order. At higher temperatures the emergence of isolated disclinations suppressed the remnant orientational order leading to a conventional, isotropic fluid. The melting of the vortex lattice in thin films of type II superconductors belong to the BKTHNY universality class. The intermediate phase is called hexatic since the orientationally-ordered liquid state is expected to have the hexagonal symmetry of the vortex lattice in the crystalline phase. In a recent paper published in Phys. Rev. Lett. we used a combination of transport measurements and STM imaging of the vortex lattice to demonstrate the existence of a hexatic phase in thin films of MoGe. Beside standard static characterization of the orientational liquid, we investigated its time evolution: by visualizing the vortex lattice at regular times steps we proved that vortexes in the hexatic fluid phase move, due to internal stress, along preferantial directions corresponding to hexgonal order.




  

Last Modified August 2019