



Next: Indirect measurements
Up: Evaluation of uncertainty: general
Previous: Evaluation of uncertainty: general
Contents
The first step consists in evaluating the uncertainty
on a quantity measured directly.
The most common likelihoods which describe the observed values
are the Gaussian, the binomial and the
Poisson distributions.
- Gaussian:
- This is the well-known case of `normally' distributed errors.
For simplicity, we will only consider
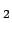 independent
of
independent
of  (constant r.m.s. error within the range of measurability),
but there is no difficulty of principle in treating the general case.
The following cases will be analysed:
(constant r.m.s. error within the range of measurability),
but there is no difficulty of principle in treating the general case.
The following cases will be analysed:
- inference on
 starting from a prior much more vague than
the width of the likelihood (Section
starting from a prior much more vague than
the width of the likelihood (Section ![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
- prior width comparable with that of the likelihood
(Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ): this case also describes the
combination of independent measurements;
): this case also describes the
combination of independent measurements;
- observed values very close to,
or beyond the edge of the physical region (Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
- a method to give unbiased estimates will be discussed
in Sections
![[*]](file:/usr/lib/latex2html/icons/crossref.png) and
and ![[*]](file:/usr/lib/latex2html/icons/crossref.png) ,
but at the cost of having to introduce
fictitious quantities.
,
but at the cost of having to introduce
fictitious quantities.
- Binomial:
- This distribution is important for efficiencies
and, in the general case, for making inferences on unknown proportions.
The cases considered include (see Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ):
):
- general case with flat prior leading to the
recursive Laplace formula (the problem solved originally by Bayes);
- limit to normality;
- combinations of different datasets coming from
the same proportion;
- upper and lower limits when the efficiency is 0 or 1;
- comparison with Poisson approximation.
- Poisson:
- The cases of counting
experiments
here considered2.15
are (see Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ):
):
- inference on
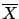 starting from a flat distribution;
starting from a flat distribution;
- upper limit in the case of null observation;
- counting measurements in the presence of a background, when its rate
is well known (Sections
![[*]](file:/usr/lib/latex2html/icons/crossref.png) and
and ![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
- more complicated case of background with an uncertain rate
(Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
- dependence of the conclusions on the choice of
experience-motivated priors (Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
- combination of upper limits, also considering
experiments of different sensitivity (Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ).
).
- effect of possible systematic errors (Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
- a special section will be dedicated to the lower bounds on the mass
of a new hypothetical particle from counting experiments
and from direct information (Section
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ).
).




Next: Indirect measurements
Up: Evaluation of uncertainty: general
Previous: Evaluation of uncertainty: general
Contents
Giulio D'Agostini
2003-05-15
 starting from a prior much more vague than
the width of the likelihood (Section
starting from a prior much more vague than
the width of the likelihood (Section ![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ): this case also describes the
combination of independent measurements;
): this case also describes the
combination of independent measurements;
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
![[*]](file:/usr/lib/latex2html/icons/crossref.png) and
and ![[*]](file:/usr/lib/latex2html/icons/crossref.png) ,
but at the cost of having to introduce
fictitious quantities.
,
but at the cost of having to introduce
fictitious quantities.
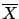 starting from a flat distribution;
starting from a flat distribution;
![[*]](file:/usr/lib/latex2html/icons/crossref.png) and
and ![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ).
).
![[*]](file:/usr/lib/latex2html/icons/crossref.png) );
);
![[*]](file:/usr/lib/latex2html/icons/crossref.png) ).
).